User:SuperSaiyan2Link/Suikoden Explosion

In the Suikoden series, there is a magic system known as Runes, which allow for characters to fight with different kinds of magic. One Rune in particular, the Fire Rune, allows for several explosions that we can use to gauge the strength of the characters.

The Fire Rune specifically has an attack called Explosion, which you can visually see here from Suikoden I, though Suikoden II's Dancing Flames attack is pretty much the exact same move, but easier to actually see visually. You can see the animation for Dancing Flames here, as it's what I'm going to use for this calc.

Also, the formula I'm going to use for this calc is this:

W = R^3*((27136*P+8649)^(1/2)/13568-93/13568)^2

W is the yield in tons in TNT, R is the radius in meters, P is the shockwave pressure in bars.

So, let's begin.

---

The brunt of the attack is when the explosion actually lands on the opponent, though the opponent quickly becomes obscured by the blast. Thankfully, there is a quick frame where we can see the character within the explosion to use as a gauge of the explosion's size:

The character within the blast here is Luca Blight, the main villain of Suikoden II. I would use the average male height, around 5'6", but Luca is usually seen as taller than most citizens, so I'm going to go with an even 6'0". Through this, we can start with the calculation.

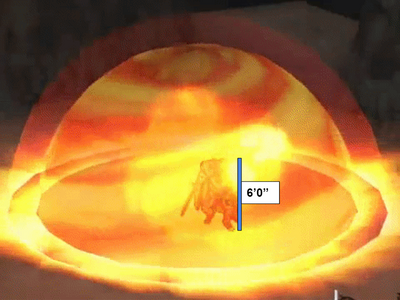
Here is Luca in comparison to the explosion. He's at the epicenter of the blast, which makes this much easier.

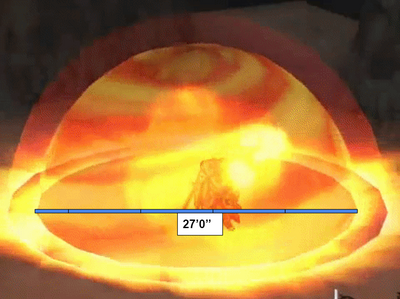
Using Luca Blight's height (heh), I found the diameter of the Dancing Flames explosion. The diameter came out to be 4½ Luca's, or 27'0". That would thus make the radius of the blast 13'6", or 4.12 meters.

R = 4.12 meters

All we really need to calculate now would be P, the shockwave pressure. Thankfully I don't need to find this one myself, since the standard amount used for all calcs is 1.37895 bars or 20 psi.

P = 1.37895 bars

With those done, I can finish the calculation and see how strong the blast is.

W = 4.12^3*((27136*1.37895+8649)^(1/2)/13568-93/13568)^2

W = 0.00562 tons

0.00562 tons = Room level (9-A)